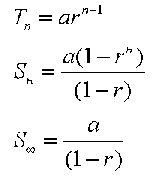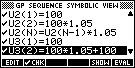GP Aplets
An easy way to solve
problems with Geometric Progressions
An easy way to solve Geometric Progression problems is to save copies of the Solve and Sequence aplets as "GP Solver" and "GP Sequence" and then pre-load them with equations which allow easy solution of many problems.
Setting up the aplets
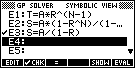
In the APLET view, RESET the Solve aplet and then SAVE it under a new name of "GP Solver" or whatever you'd like to call it. START the Aplet and enter the three equations for Geometric
sequences shown below. Note that you MUST put a times sign (*) after the A
in the second equation. An explanation of why this is necessary can
be found elsewhere.
|

|
These equations can be used to solve a typical GP problem. For
example...
For the sequence defined by |
|
| (i) CHK the first equation E1 and then change to the
NUM view. Enter the values of N=20, A=3 and R=1.4.
Press SOLVE on T. Answer: 1792.89 (to 2 d.p.) |
 |
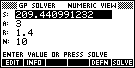
| (ii) CHK the second equation E2 and then
change to the NUM view again. Update the value of N to 10 and
then SOLVE on S. Answer: 209.44 |
 |
| (iii) There is no need to use E3 in this case. Since the ratio is greater than 1, the sum to infinity will not exist. It is important to note that the aplet would still give you an (incorrect) answer! There is no substitute for using your brain. | |
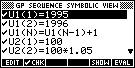
Now do the same to the Sequence aplet, saving it as
"GP Sequence" and entering the equations shown
right. The particular equations shown are for the problem shown
below, and will need to be modified when other problems are to be solved.In 1995 Fred deposited $100 into a bank account paying 5% compound interest p.a. calculated annually. His brother Jim made the same deposit but he also added to the account regularly by depositing another $100 at the end of each year (just after the interest is calculated). |
|
| If you now change to the NUM view you will see that it is ideally set up to solve this problem. The U1 column shows the year, the U2 column shows Fred's balance and the U3 column shows Jim's. | 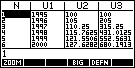 |
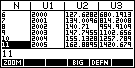
Scrolling down to 2005, we can see that Fred has $162.89, while Jim has $1420.68 |
 |
| Continuing to scroll down, we can see that Fred's balance exceeds $1000 for the first time at the start of 2043. Remember that the values represent the account balances at the start of each year. | 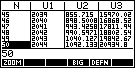 |
| Moving backwards, we can see that Jim's balance in 2020 was $5111.35 Since this is T26, Jim has made 26 deposits of $100. His profit is therefore $5111.35 - 26*$100 which is $2511.35 | 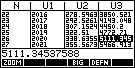 |